PROFIT FUNCTION
Given any production set Y, we have seen how to calculate the
profit function. 7г(р), which gives us the maximum profit
attainable at prices p. The profit function possesses several important
properties that follow directly from its definition. These properties are very
useful for analyzing profit-maximizing behavior.
Recall that the profit function is, by definition, the maximum profits
the firm can make as a function of the vector of prices of the net outputs:
7г(р) = max py у
such that у is in Y.
					







From the viewpoint of the mathematical results that follow, what is
important is that the objective function in this problem is a linear function
of prices.
Properties of the profit function
We begin by outlining the properties of the profit function. It is
important to recognize that these properties follow solely from the assumption
of profit maximization. No assumptions about convexity, monotonicity, or other
sorts of regularity are necessary.
Properties of the profit function
1) 
Nondecreasing
in output prices, nonincreasing in input prices. If’ p\ > pi for all outputs
and p«- < pj for all inputs, then 7r(p») > 7r(p).
2) 
Homogeneous
of degree 1 in p. 7r(£p) — £тг(р) for all t > 0.
3) 
Convex
in p. Let
p« = fp + (1 — t)p« for 0 < t < 1. Then тг(р») < *7г(р) + (1-*)тг(р»).
4)Continuous in p. The function ir(p)
is continuous, at least when 7r(p) is well-defined and pt >
0 for i = 1,…, n.
Proof. We emphasize once more that the proofs of these properties follow from
the definition of the profit function alone and do not rely on any properties
of the technology.
1)
Let у be a profit-maximizing net output vector at
p, so that 7r(p) = py and let y« be a profit-maximizing net output vector at p»
so that 7r(p«) = p»y«. Then by definition of profit maximization we have p»y’
> p«y. Since p»i > Pi for all i for which j/i > 0
and p\ < pi for all i for which y^ < 0, we also have
p«y > py. Putting these two inequalities together, we have » (p») = р«у» > РУ = it(p),
as required.
2) Let у be a profit-maximizing net output
vector at p, so that py > py« for all y» in Y. It follows that for t
> 0, tpy > tpy« for all y» in Y. Hence у also maximizes profits at prices tp.
Thus ir(tp) = tpy = tn(p).
3) Let у maximize profits at p, y’ maximize
profits at p’, and y« maximize profits at p». Then we have
тг(р»)
= p«y» = (tp + (1 — t)p«)y« = tpy» + (1 — t)p»y«. (3.1)
By the definition of profit maximization, we know that
фу» < Фу = Мр)
(l-t)p«y»<(l-t)p»y« = (l-t)n(p»). Adding these two
inequalities and using (3.1). we have
TT(p»)<tTr(p) + (l-t)7T(p’).
as required.
4) The continuity of 7r(p) follows from the Theorem of the
Maximum de scribed in Chapter 27. page 506. I
The facts that the profit function is homogeneous of degree 1 and
increasing in output prices are not terribly surprising. The convexity
property, on the other hand, does not appear to be especially intuitive.
Despite this appearance there is a sound economic rationale for the convexity
result, which turns out to have very important consequences.
Consider the graph of profits versus the price of a single output good,
with the factor prices held constant, as depicted in Figure 3.1. At the price
vector (p*,w*) the profit-maximizing production plan (y*,x*) yields profits p*y*
— w*x*. Suppose that p increases, but the firm continues to use the same
production plan (y*,x.*). Call the profits yielded by this passive
behavior the «passive profit function» and denote it by П(р) = py* — w*x*. This is easily seen to be a straight line. The
profits from pursuing an optimal policy must be at least as large as the
profits from pursuing the passive policy, so the graph of ir(p) must lie
above the graph of H(p). The same argument can be repeated for any price
p, so the profit function must lie above its tangent lines at every
point. It follows that n(p) must be a convex function.











PROFITS

MP)




J U(p)


= py-


— w«x»




MP’)




^j^\




S^ P*

OUTPUT PRICE




Figure The profit function. As the output price increases, the profit 3.1 function
increases at an increasing rate.
The properties of the profit function have several uses. At this point we
will satisfy ourselves with the observation that these properties offer several
observable implications of profit-maximizing behavior. For example,
suppose that we have access to accounting data for some firm and observe that
when all prices are scaled up by some factor t > 0 profits do not
scale up proportionally. If there were no other apparent changes in the
environment, we might suspect that the firm in question is not maximizing profits.
EXAMPLE: The effects of price stabilization
Suppose that a competitive industry faces a randomly fluctuating price
for its output. For simplicity we imagine that the price of output will be pi
with probability q and pi with probability (1 — q). It
has been suggested that it may be desirable to stabilize the price of output at
the average price P — 4P\ + (1 ~ 4)P2- How would this affect
profits of a typical firm in the industry?
We have to compare average profits when p fluctuates to the
profits at the average price. Since the profit function is convex,
Qir(pi) + (1 — 9)t(P2) > f(<7Pi
+ (1 — q)P2) = 7
Thus average profits with a fluctuating price are at least as large as
with a stabilized price.
At first this result seems counterintuitive, but when we remember the
economic reason for the convexity of the profit function it becomes clear. Each
firm will produce more output when the price is high and less when the price is
low. The profit from doing this will exceed the profits from producing a fixed
amount of output at the average price.
Supply and demand functions from the profit function
If we are given the net supply function y(p), it is easy to calculate the
profit function. We just substitute into the definition of profits to find
t(p) = РУ(Р)-
Suppose that instead we are given the profit function and are asked to
find the net supply functions. How can that be done? It turns out that there is
a very simple way to solve this problem: just differentiate the profit
function. The proof that this works is the content of the next proposition.
Hotelling«s lemma. (The derivative property) Let j/j(p) be the firm»s net supply
function for good i. Then
Vi\V = —r for г = l,…,n,
OPi
assuming that the derivative exists and that pi > 0.
Proof. Suppose (y*) is a profit-maximizing net output vector at prices (p*).
Then define the function
g(p) = тг(р) -ру*.
Clearly, the profit-maximizing production plan at prices p will always be
at least as profitable as the production plan y*. However, the plan y* will be
a profit-maximizing plan at prices p*, so the function g reaches a
minimum value of 0 at p*. The assumptions on prices imply this is an interior
minimum.
The first-order conditions for a minimum then imply that
дд(р*)
Этг(р*) 
—£ = —и Vi = 0 for г
= 1,…, п.
dpi дрг
Since this is true for all choices of p*, the proof is done. I
The above proof is just an algebraic version of the relationships
depicted in Figure 3.1. Since the graph of the «passive» profit line
lies below the graph of the profit function and coincides at one point, the two
lines must be tangent at that point. But this implies that the derivative of
the profit function at p* must equal the profit-maximizing factor supply
at that price: y(p*) = дтт(р*)/др.
The argument given for the derivative property is convincing (I hope!)
but it may not be enlightening. The following argument may help to see what is
going on.
Let us consider the case of a single output and a single input. In this
case the first-order condition for a maximum profit takes the simple form
/Ш _ w = o. (3.2)
ax
The factor demand function x(p, w) must satisfy this first-order
condition. The profit function is given by
7r(p, w) = pf(x(p, w)) — Wx(p, w).
Differentiating the profit function with respect to w, say, we
have
дтт df(x(p,w)) дх дх
-к- =
Р я я w— x(p, w)
aw ox ow aw
df{x(p,w))
P я w
ox
Ox
Substituting from (3.2), we see that
дтт
— = -x(p,u,)-
The minus sign comes from the fact that we are increasing the price of an
input—so profits must decrease.
This argument exhibits the economic rationale behind Hotelling’s lemma.
When the price of an output increases by a small amount there will be two
effects. First, there is a direct effect: because of the price increase the
firm will make more profits, even if it continues to produce the same level of
output.
But secondly, there will be an indirect effect: the increase in the
output price will induce the firm to change its level of output by a small
amount. However, the change in profits resulting from any infinitesimal change in
output must be zero since we are already at the profit-maximizing production
plan. Hence, the impact of the indirect effect is zero, and we are left only
with the direct effect.
The envelope theorem
The derivative property of the profit function is a special case of a
more general result known as the envelope theorem, described in Chapter
27, page 491. Consider an arbitrary maximization problem where the objective
function depends on some parameter a:
M(a) = max f(x,a).
X
The function M(a) gives the maximized value of the objective
function as a function of the parameter a. In the case of the profit
function a would be some price, x would be some factor demand,
and M(a) would be the maximized value of profits as a function of the
price.
Let x(a) be the value of x that solves the maximization
problem. Then we can also write M(a) = f(x(a), a). This simply says that
the optimized value of the function is equal to the function evaluated at the
optimizing choice.
It is often of interest to know how M(a) changes as a changes.
The envelope theorem tells us the answer:
dM(a) <9/(x, a)
da da=x(a)
This expression says that the derivative of M with respect to a
is given by the partial derivative of / with respect to a, holding x
fixed at the optimal choice. This is the meaning of the vertical bar to the
right of the derivative. The proof of the envelope theorem is a relatively
straightforward calculation given in Chapter 27, page 491. (You should try to
prove the result yourself before you look at the answer.)
Let’s see how the envelope theorem works in the case of a simple
one-input, one-output profit maximization problem. The profit maximization
problem is
7r(p, w) = max pf(x) — wx.
46 PROFIT FUNCTION (Ch. 3)
The a in the envelope theorem is p or w, and M(a)
is n(p, w). According to the envelope theorem, the derivative of
7r(p, w) with respect to p is simply the partial derivative
of the objective function, evaluated at the optimal choice:
9^1 =f(X)\ = /(*(*«,)).
Op x=x(p,w)
This is simply the profit-maximizing supply of the firm at prices (p,
w).
Similarly,
dn{p, w)
—я = ~x =-x(p,w),
aw x=x(p,w)
which is the profit-maximizing net supply of the factor.
Comparative statics using the profit function
At the beginning of this chapter we proved that the profit function must
satisfy certain properties. We have just seen that the net supply functions are
the derivatives of the profit function. It is of interest to see what the
properties of the profit function imply about the properties of the net supply
functions. Let us examine the properties one by one.
First, the profit function is a monotonic function of the prices. Hence,
the partial derivative of 7r(p) with respect to price i will be negative
if good i is an input and positive if good i is an output. This
is simply the sign convention for net supplies that we have adopted.
Second, the profit function is homogeneous of degree 1 in the prices. We
have seen that this implies that the partial derivatives of the profit function
must be homogeneous of degree 0. Scaling all prices by a positive factor t won’t
change the optimal choice of the firm, and therefore profits will scale by the
same factor t.
Third, the profit function is a convex function of p. Hence, the matrix
of second derivatives of 7r with respect to p—the Hessian matrix—must be a
positive semidefinite matrix. But the matrix of second derivatives of the
profit function is just the matrix of first derivatives of the net
supply functions. In the two-good case, for example, we have




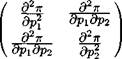
(dy,дш\
= dpidp2
I dy2dy2 I «
\ dp{op2 ‘
The matrix on the right is just the substitution matrix—how the net
supply of good г changes as the price of good j changes.
It follows from the properties of the profit function that this must be a
symmetric, positive semidefinite matrix.
The fact that the net supply functions are the derivatives of the profit
function gives us a handy way to move between properties of the profit function
and properties of the net supply functions. Many propositions about
profit-maximizing behavior become much easier to derive by using this
relationship.
EXAMPLE: The LeChatelier principle
Let us consider the short-run response of a firm’s supply behavior as
compared to the long-run response. It seems plausible that the firm will
respond more to a price change in the long run since, by definition, it has
more factors to adjust in the long run than in the short run. This intuitive
proposition can be proved rigorously.
For simplicity, we suppose that there is only one output and that the
input prices are all fixed. Hence the profit function only depends on the
(scalar) price of output. Denote the short-run profit function by ns(p,z) where
z is some factor that is fixed in the short run. Let the long-run
profit-maximizing demand for this factor be given by z(p) so that the
long-run profit function is given by itl(p)
= ^s{Pi z{p))- Finally, let p* be some
given output price, and let z* = z(p*) be the optimal long-run demand
for the 2-factor at p*.
The long-run profits are always at least as large as the short-run
profits since the set of factors that can be adjusted in the long run includes
the subset of factors that can be adjusted in the short run. It follows that
h(p) = nL(p) — ns(p, 2*) = ns(p, z(p))  —
7TS(p, z*) > 0
for all prices p. At the price p* the difference between
the short-run and long-run profits is zero, so that h(p) reaches a
minimum at p = p*. Hence, the first derivative must vanish at p*. By
Hotelling’s lemma, we see that the short-run and the long-run net supplies for
each good must be equal at p*.
But we can say more. Since p* is in fact a minimum of h(p),
the second derivative of h(p) is nonnegative. This means that
d2nL(p*) d2ns(p*,z*) >Q
dp2 dp2
~
Using Hotelling’s lemma once more, it follows that
dVLJP*) dys(p*,z*) = d2irL{p*)
d2ns(p*,z*) >Q
dp dp dp2
dp2 ~
This expression implies that the long-run supply response to a change in
price is at least as large as the short-run supply response at z* = z(p*).
Notes
The properties of the profit function were developed by Hotelling (1932),
Hicks (1946), and Samuelson (1947).